The quadratic mapping is given by \begin{equation}\overset { o }{ \varphi } ={ \overset { o }{ \varphi } }_{ 0 }^{ 2 }+\overset { o }{ c } ,\end{equation} where the variable $\overset { o }{ \varphi } $ and the constant $\overset { o }{ c } $ are scator elements. The recurrence relationship is \begin{equation} \overset { o }{ \varphi }_{ n+1 } ={ \overset { o }{ \varphi } }_{ n }^{ 2 }+\overset { o }{ c }, \end{equation} where the subindex indicates the iteration number.
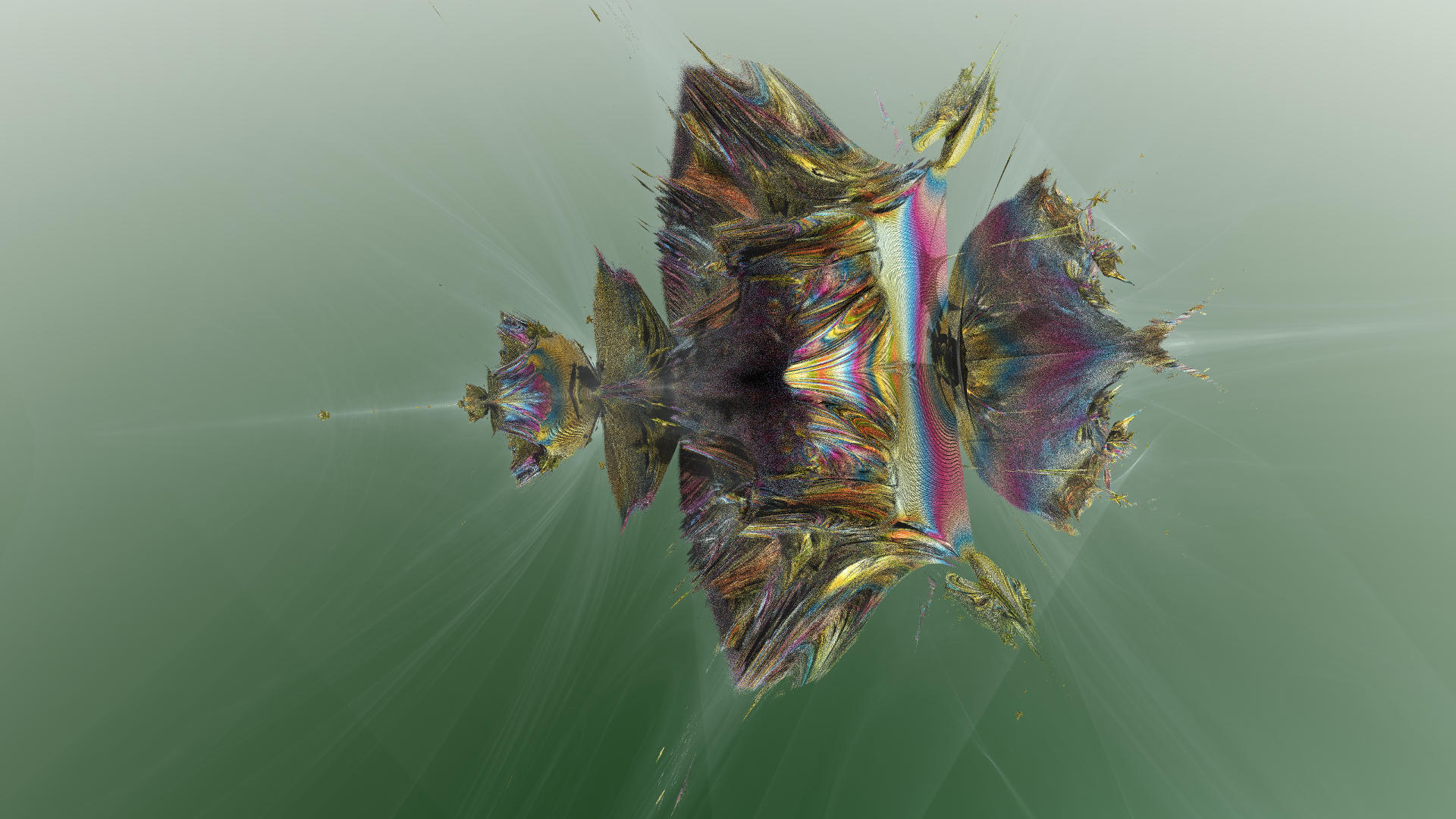
dynamical space
Consider a constant point in hyper complex space, say $\overset{o}{c}_{0}$. Commence with any other point in hypercomplex space $\overset{o}{\zeta}_{1}$. Evaluate the square of this latter point plus the constant point. Perform this quadratic mapping repeatedly $ \overset{o}{\zeta}_{n+1}=\overset{o}{\zeta}_{n}^{2}+\overset{o}{c}_{0} $. The first few iterations are
\begin{equation} \overset{o}{\zeta}_{2} = \overset{o}{\zeta}_{1}^{2}+\overset{o}{c}_{0}\end{equation}
\begin{equation} \overset{o}{\zeta}_{3} = \left(\overset{o}{\zeta}_{1}^{2}+\overset{o}{c}_{0}\right)^{2}+\overset{o}{c}_{0}\end{equation}
\begin{equation} \overset{o}{\zeta}_{4} = \left(\left(\overset{o}{\zeta}_{1}^{2}+\overset{o}{c}_{0}\right)^{2}+\overset{o}{c}_{0}\right)^{2}+\overset{o}{c}_{0}\end{equation}
Evaluation is performed for a number of representative points $\overset{o}{\zeta}$ in the hypercomplex space. The magnitude of the outcome is calculated after each iteration, and in particular, whether the magnitude after many iterations diverges or remains bounded.
The points in hypercomplex space that do not diverge after an infinite number of iterations belong to the imaginary scators bound set under the quadratic iteration. There is a bound set in hypercomplex space for each constant $\overset{o}{c}_{0}$. Each point in hypercomplex space has, so to speak, a fingerprint.
Points that do not lie in the boundary between bound and divergent points belong to the Fatou set. In contrast, points in the boundary, have a vicinity where some of the points diverge and some others remain bounded. Points that do not belong to the Fatou set, belong to the Julia set. The points at the boundary are elements of the Julia set.
The filled in Julia set is made up by the points that do not diverge, that is, this set contains the Fatou set points that do not tend to infinity. This set is equal to the bound set under the quadratic mapping. The boundary between points that diverge and those that do not is extremely intricate. Point that diverge can be coloured depending on how fast they diverge under the iteration process.
If the initial point is $\overset{o}{\zeta}_{1}=\overset{o}{c}_{0}$, then the point is part of the iteration in the Julia scheme (dynamical space) as well as the Mandelbrot scheme (parameter space).
the origin
If the hyper complex space initial point is zero $\overset{o}{c}_{0}=0$, after $n$ iterations \[ \overset{o}{\zeta}_{n}=\overset{o}{\zeta}_{1}^{2n-1} \]
In the multiplicative representation, a scator number with magnitude greater than one diverges, while a number with magnitude less than one converges to zero. {comentar si son imaginarios o reales} That is, the bound set is a cusphere with unit 'radius'. Points within the cusphere get closer to the origin while points outside the unit cusphere tend to infinity. The boundary is the surface with constant magnitude equal to one.
The fingerprint of zero is the bound set in dynamical space under the quadratic iteration (Julia set in hypercomplex space). The boundary of this set is identical to the unit magnitude scator set. Therefore:
The fingerprint of zero is the unit magnitude scator set.
parameter space
In parameter space, the iteration always begins with zero initial value $\overset{o}{\zeta}=0$. The first few iterations of $\overset{o}{\zeta}$ are
\begin{equation}\overset{o}{\zeta}_{1} = 0\end{equation}
\begin{equation}\overset{o}{\zeta}_{2} = \overset{o}{c}_{0}\end{equation}
\begin{equation}\overset{o}{\zeta}_{3} = \overset{o}{c}_{0}^{2}+ \overset{o}{c}_{0}\end{equation}
\begin{equation}\overset{o}{\zeta}_{4} = \left(\overset{o}{c}_{0}^{2}+\overset{o}{c}_{0}\right)^{2}+\overset{o}{c}_{0}\end{equation}
The points in hyper complex space that do not diverge after an infinite number of iterations belong to the scators bound set under the quadratic iteration. These sets are a generalization of the Mandelbrot set or M-set. {comentar si son imaginarios o reales}

