Imaginary scator algebra is a non distributive extension of complex algebra to higher dimensions. The square of a scator $\overset{o}{\varphi}=s+x\check{\mathbf{e}}_{x}+y\check{\mathbf{e}}_{y}$, is
\begin{equation} \overset{o}{\varphi}^{2}=s_{\diamond}+x_{\diamond}\check{\mathbf{e}}_{x}+y_{\diamond}\check{\mathbf{e}}_{y}=s^{2}\left(1-\frac{x^{2}}{s^{2}}\right)\left(1-\frac{y^{2}}{s^{2}}\right)+2sx\left(1-\frac{y^{2}}{s^{2}}\right)\check{\mathbf{e}}_{x}+2sy\left(1-\frac{x^{2}}{s^{2}}\right)\check{\mathbf{e}}_{y}.\label{eq:sca squ fact} \end{equation}
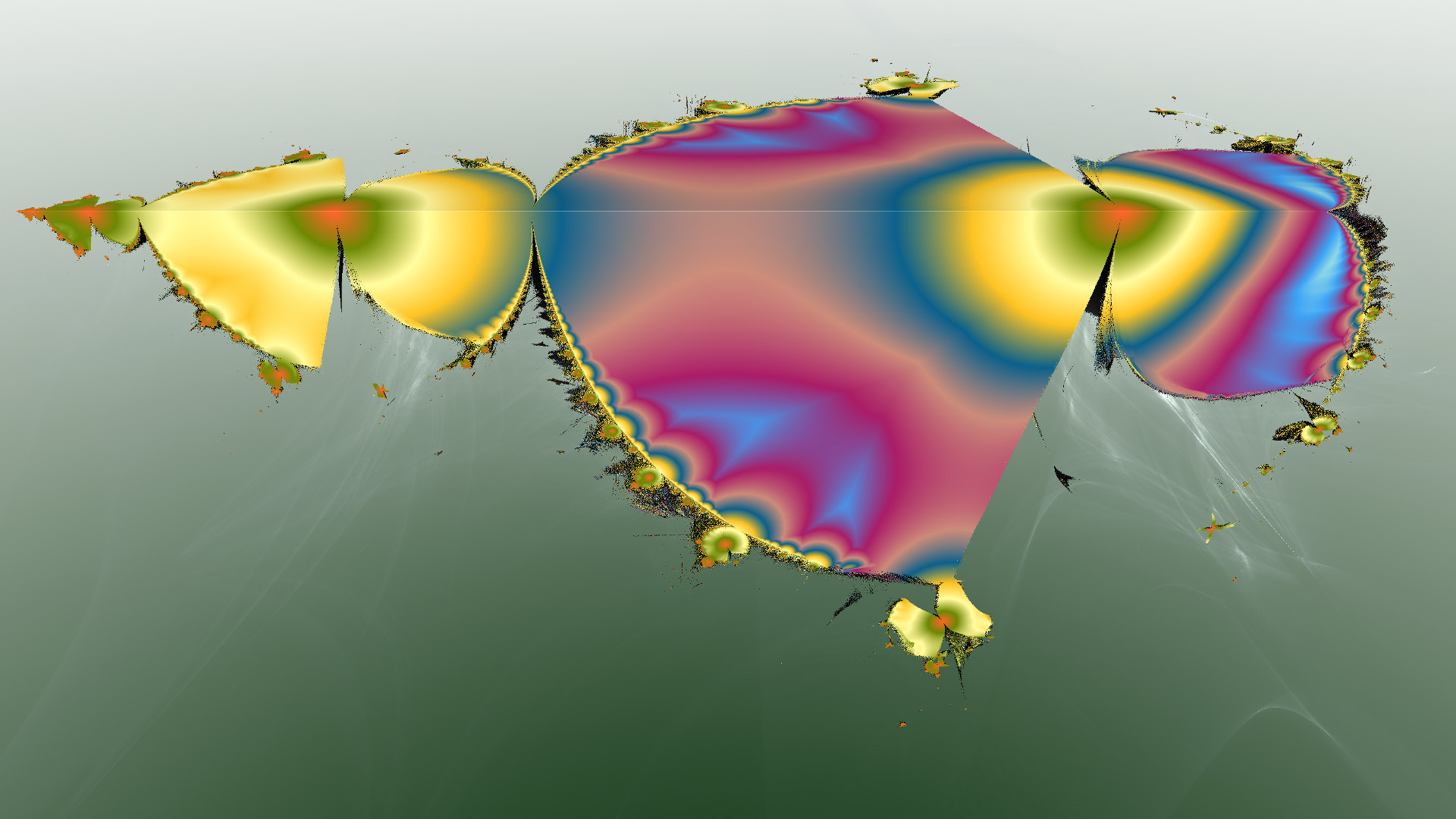
The quadratic mapping for the scalar component in imaginary scator algebra is,
\begin{equation} s_{m+1}=s_{m}^{2}\left(1-\frac{x_{m}^{2}}{s_{m}^{2}}\right)\left(1-\frac{y_{m}^{2}}{s_{m}^{2}}\right)+s,\label{eq:iter scalar} \end{equation}
and for the director components, the recurrence relationship is
\begin{equation} x_{m+1}=2s_{m}x_{m}\left(1-\frac{y_{m}^{2}}{s_{m}^{2}}\right)+x,\label{eq:iter dir1} \end{equation}
\begin{equation} y_{m+1}=2s_{m}y_{m}\left(1-\frac{x_{m}^{2}}{s_{m}^{2}}\right)+y.\label{eq:iter dir2} \end{equation}
The square magnitude of an imaginary scator $\overset{o}{\varphi}$ is
\begin{equation} \bigl\Vert\overset{o}{\varphi}\bigr\Vert^{2}=\overset{o}{\varphi}\overset{o}{\varphi}^{*}=s^{2}+x^{2}+y^{2}+\frac{x^{2}y^{2}}{s^{2}}.\label{eq:sca mag squ} \end{equation}
With this preamble of imaginary scator algebra, it is then possible to perform the quadratic iteration. It is then possible to visualize the bound sets in two or three dimensions as well as the escape velocities of unbound points.
An introductory article about fractals with imaginary scators can be obtained in the following link http://www.worldscientific.com/doi/pdf/10.1142/S0218127416300020

